Next: The Geodesic Equation Up: A Little General Relativity Previous: Gravity's Effect on Time Contents
We know that since photons are massless,
![]() .
Consider a photon moving outward radially.
We can compute the radial velocity in the Schwarzschild coordinates.
.
Consider a photon moving outward radially.
We can compute the radial velocity in the Schwarzschild coordinates.
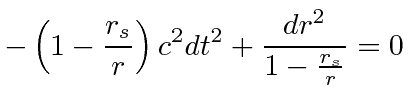 |
||
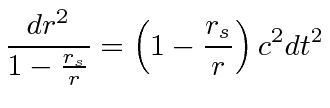 |
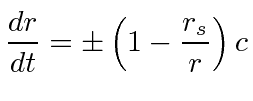 |
 , the velocity is zero and the photon does not come out.
This is consistent with our redshift result which says the frequency will be red shifted to zero
for a photon emitted from exactly the Schwarzschild radius.
, the velocity is zero and the photon does not come out.
This is consistent with our redshift result which says the frequency will be red shifted to zero
for a photon emitted from exactly the Schwarzschild radius.
This solution in the normal spherical coordinates has a singularity.
It is unclear what happens for
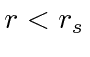 .
When analyzed in coordinates without this singularity, we find that photons will be pulled to
.
When analyzed in coordinates without this singularity, we find that photons will be pulled to
![]() if they
start out with
if they
start out with
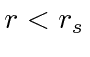 .
This is of course the phenomenon of the
Black Hole.
No light can come out.
.
This is of course the phenomenon of the
Black Hole.
No light can come out.
A collapsing star is seen from the outside to take an infinite amount of time to collapse down to the Schwartzchild radius due to the gravitational effect on time. So, from the outside, a black hole is matter nearly at the Schwartzchild radius, plus some matter that was already inside that radius when the star collpsed. According to GR, the black hole collapses down to a point, but we have no way of ever measuring that from the outside.
Jim Branson 2012-10-21